Решить логарифмическое неравенство с ОДЗ

Question

Решить логарифмическое неравенство с ОДЗ

---

Answer 1

ОДЗ: 0\\ \frac{2x+2}{5x-1} \neq 1\\ 5x-1\neq 0\\ 10x^2+x-2>0 \end{cases}\end{equation*} \Rightarrow \begin{equation*}\begin{cases} x\in(-\infty; -1)\cup(0.2; +\infty)\\ x \neq 1\\ x\neq 0.2\\ x\in(-\infty; -0.5)\cup(0.4; +\infty) \end{cases}\end{equation*}\Rightarrow\\ \Rightarrow x\in(-\infty; -1)\cup(0.4; 1)\cup(1; +\infty)" alt="\begin{equation*}\begin{cases} \frac{2x+2}{5x-1}>0\\ \frac{2x+2}{5x-1} \neq 1\\ 5x-1\neq 0\\ 10x^2+x-2>0 \end{cases}\end{equation*} \Rightarrow \begin{equation*}\begin{cases} x\in(-\infty; -1)\cup(0.2; +\infty)\\ x \neq 1\\ x\neq 0.2\\ x\in(-\infty; -0.5)\cup(0.4; +\infty) \end{cases}\end{equation*}\Rightarrow\\ \Rightarrow x\in(-\infty; -1)\cup(0.4; 1)\cup(1; +\infty)" align="absmiddle" class="latex-formula">

Воспользуемся следующим методом рационализации:

В частности, так как ,

Тогда исходное неравенство станет равносильным неравенству:

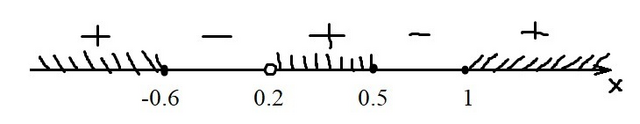
Решим неравенство методом интервалов (см. рис.). Получим, что

Объединяя полученный промежуток с ОДЗ, получим ответ.

Ответ:

---